Have you ever though about how far you can shoot a champagne cork? The swedish physicist Hans-Uno Bengtsson has actually done the necessary calculations in the wonderful Swedish book “Kring flaskor och fysik” (which translates to something like “Among bottles and physics”, it was written together with sommelier Mischa Billing). Assuming a bottle pressure of 6 atmospheres, a cork length of 25 mm (the part in contact with the bottle), a radius of 9 mm and a mass of 7.5 g, this gives an initial cork velocity of approximately 20 meters per second or 70 km/h! This translates into a maximum shot length of around 40 m (if we neglect air resistance). In case you prefer not to shoot the cork, you could of coarse turn to a saber or a heavy kitchen knife instead to open the bottle.
When opening a bottle of champagne, you might have noticed the cloud forming right above the bottle neck (see picture below). This is due to a significant temperature drop, caused by gas expansion when we open the bottle. Assuming an adiabatic expansion (meaning no heat exchange with the surroundings), Hans-Uno Bengtsson has calculated a temperature drop of 112 °C! No wonder the vapor around the bottle neck immediately freezes forming a small cloud.

(picture by polarunner at flickr.com)
If this doesn’t satisfy your craving for champagne science, there’s a whole book on the subject: “Uncorked – The Science of Champagne” by Gérard Liger-Belair. He’s an associate professor of physical sciences at the University of Reims Champagne-Ardenne and probably knows more about champagne bubbles than anyone else! In addition to many fascinating pictures of bubbles, the book has many interesting facts. Did you know that:
(these facts should be perfect conversation starters!)
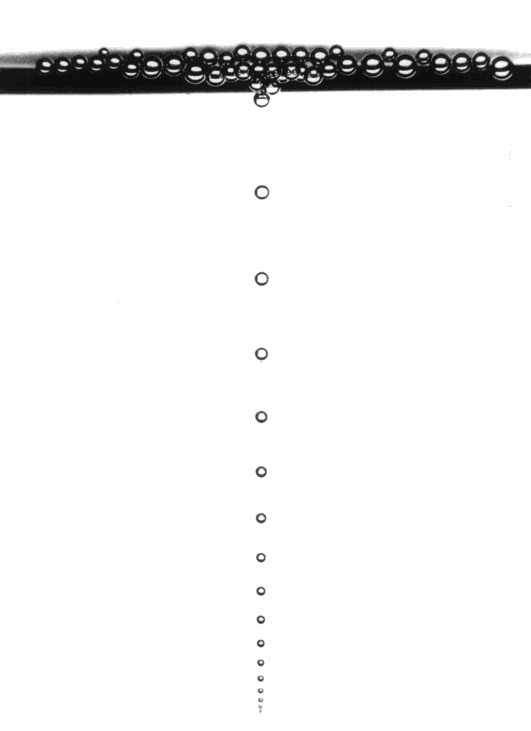
(photo by Gérard Liger-Belair)
An interesting article by Gérard Liger-Belair, “Effervescence in a glass of champagne: A bubble story” is available from Europhysics news.
Happy New Year!

I’ve performed he same kind of computation for the speed of a champagne cork (in french, sorry ; for those who can read it it’s here : http://eric.cabrol.free.fr/dotclear/index.php/2006/12/11/384-plop)
I find a velocity quite superior to the one you give here : 5 atm (relative pressure) on a diameter of 25 mm (area ~ 5 cm²) gives a force of 250 N.
Assuming a weight of 10g, the resulting acceleration is 25000 m/s².
After 0.002s the cork velocity is 50m/s, and it is only 5cm away from the bottle (so we can guess the pressure is still acting on it, although with rapidly decreasing intensity) …
The friction force f = deltaP * pi * r^2 where deltaP is pressure difference between atmosphere and bottle and r is radius of cork. Hans-Uno Bengtsson considers the fact that the friction changes as the cork leaves the flask. He sets up an integral for the work which turns out to be one half times the friction force times the length of the cork. This rearranges to the following:
v = sqrt ((deltaP * pi * r^2 * l)/m)
where deltaP = 5 atm, r = 9 mm, l = 25 mm, m = 7.5 g. By converting to the SI units and inserting this into the formula, he gets an initial velocity of v = 20 m/s or 70 km/h.